Risk analysis of Lookback options
Lookback options as many of you would already know are path dependant options whose payoff depends on the maximum or the minimum value of the underlier (depending on whether a call or a put) attained during the life of the option. The most basic Lookback options include the floating strike and the fixed strike (payoffs given below). More exotic lookbacks would include discrete monitoring, lookback features within other products, asianing lookback observations and so on and so forth. In this article we present the analysis of the risk factors of the most basic payoffs which could be extended (hopefully!!) to the more advance variations. Lets begin with the payoffs first.
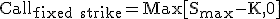
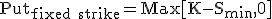
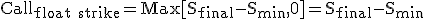
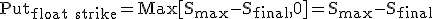
Trade motivation
An investor in stocks is always in a dilemma whether to sell and book the current profit or hold and hopefully earn still higher profits in future while risking loosing some or all of the unrealized profits.The investor wants to avoid the feeling of REGRET - the regret of holding for too long or the regret of selling too early. As it turns out, the lookback options are just the options that act as insurance against regret. For example, with floating strike calls the investor can buy the stock at the minimum price during the life of the lookback option and with floating strike puts the investor can sell at the stock at the maximum price during the life of the lookback option.
Let elaborate one example - Skip this section if you already have guessed it. Suppose I own a stock which currently trades at 150. I had originally bought that stock for 100. I have the option of selling it at 150 now and booking the profit of 50. If however I do so and the stock goes to 200, I will regret having sold it and on the othe hand I dont sell and the stock crashes to 90, I loose all the unrealized profit. So what do I do? I buy a floating strike put (ofcourse you have to pay a premium for this). Lets say the stock ends at an even stevens 100 i.e. no profit no loss. If the stock did however reach a maximum of 200 during the life of the put option. The buyer receives a payoff of 100 from the lookback put and likewise if indeed the stock crashed after reaching 150 and ends at 90, the buyer receives the payoff of 60 from the lookback put. In the first case, he earns 0 from the position in stock and 100 from the lookback put that is net 100 profit which is the maximum he could have got. In the second case, he gains 60 on the put and looses 10 on the stock making the net profit 50 which is again the maximum he could have got.
Risk analysis
Lets first consider the Fixed Strike Lookback Call. The payoff is shown again below for handiness purposes.
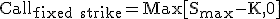
The first thing we notice is that the payoff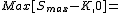 
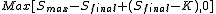is greater than 
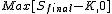which is the payoff of classical European call option. Hence this option should be costlier than a European call and as a rule of thumb mostly twice as costly as the the European calls.
We also realize that whenever the underlying stock reaches a new high (since option start date), the option locks in a payoff =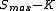 
. This is the minimum the option buyer receives no matter where the stock ends at the expiry date.
The option buyer clearly benefits from higher stock prices i.e it is long delta, long interest rates and short dividends. The payoff also benefits from higher volatility (much like a simple call and additionally, the volatility helps attain higher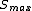 
on the upside and the downside being floored leads to a still higher fair value) meaning that the option buyer is also long volatility.
The hedging argument - (read Goldman, Sosin, and Gatto (1979) or Gatheral's book, The volatility surface). Suppose I have sold a lookback call that pays 
, to hedge the short position in such an option I'll buy two ATM european call options with strike K. If the underlier crashes and never reaches K, both the short position in the lookback option and the two european calls expire worthless so that I need not do anything else to hedge. If however, the underlier rallies and the reaches 
. Notice that that the 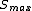is now and the option has already locked in the payoff 
. I sell the two european calls that I own and but two new ATM European call i.e I sell a call spread (aha!! the skew exposure). We know that the delta of the ATM call option is approximately 0.5 so that when the underlier moves by , the option price moves by approximately 
. Hence we are hedging using two options.
As a result of the above argurment, the seller is long skew and the buyer is short skew. Higher the skew, more valuable will be the call spread and the seller gets more money by selling it. We can conclude that when priced under local volatility, this lookback option will be overpriced. We should be using stochastic volatility for valuing this option.
Gamma is also an important risk for lookbacks. For these fixed strike calls, we observe that the gamma is maximum when the underlier is at its high and tends to come down when the underlier declines away from the high.
Lets take the Fixed Strike Lookback Put options now. The payoff is given below:
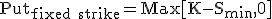
This lookback tends to lock in payoff =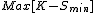 
whenever the underlying stock reaches a new low. This is the minimum no matter what path the stock follows thereafter.
The option buyer is short delta, short interest rates and long dividends. The payoff again benefits from higher volatility and hence the buyer is long volatility. For hedging, the seller will but two ATM European puts initially. If the stock rises both the lookback put and the european puts both expire worthless. If the stock declines to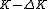 
, the seller would sell the two ATM european puts he holds and buys two new ATM puts with strike 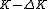i.e he is buying put spreads. In the presence of skew the 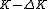puts that the seller buys to hedge would be costlier and hence he is short skew which means that the buyer is long skew.
The gamma for these lookback puts is highest when the stock is at its lowest and reduces as the stock rises.
For Floating strike Lookback calls, the payoff is:
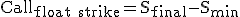
It helps to compare these lookbacks against ATM (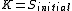
)European calls. The expected payoff of these lookback options is higher than ATM european calls as the 
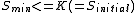making it more expensive than a European ATM call. When the underlier is at or near its low, these lookbacks would behave like ATM calls and when the stock rises higher these options would start behaving like ITM calls.
The buyer is long delta (the delta picks as the stock rises above the minimum) - closer to stock's minimum the delta would be around 0.5 and would rise to 1 as the underlying stock rises to its maximum.
He is also long volatility since higher volatility would pull apart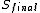 
and 
leading to higher payoffs. Gamma is maximum when the stock is at the lowest and decreases as the stock rises. The buyer is long skew (argument is similar to lookback fixed strike puts above)
Floating strike Lookback puts, the payoff is:
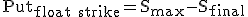
The arguments pretty much remain on the same lines as the discussion so far. Let me just quickly summarize and wrap up this article. The buyer is short delta, long volatility, the gamma is maximum when the underlying stock is at it maximum and finally the buyer is also short skew.
